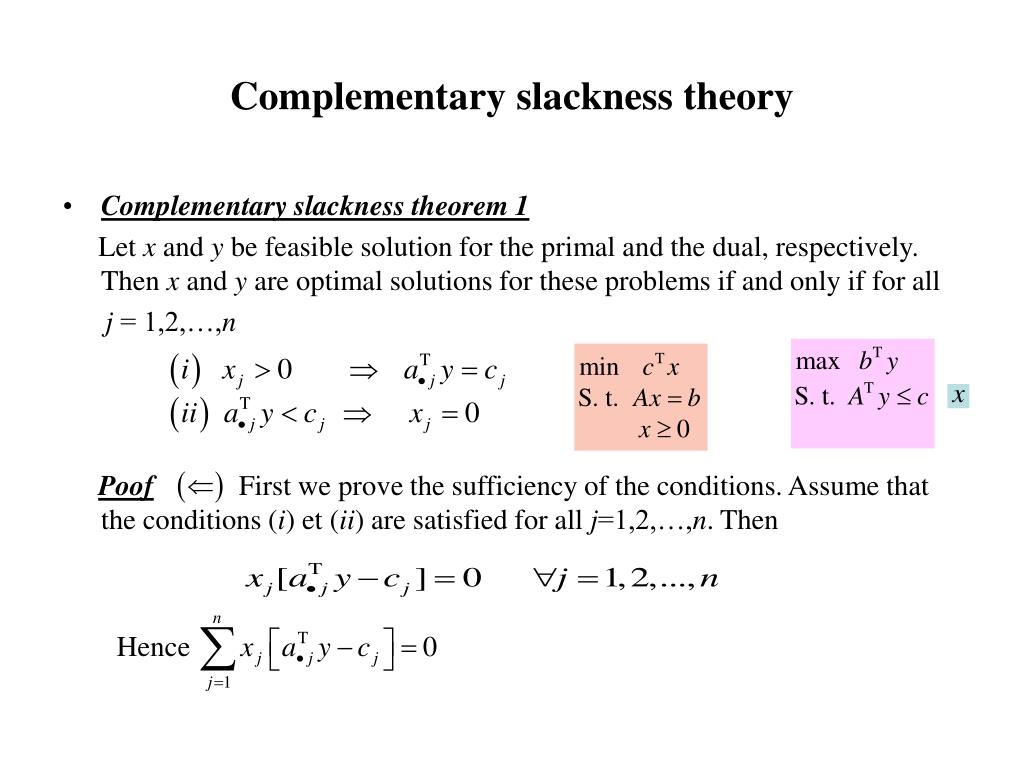
Complementary Slackness Condition
Complementary Slackness Condition - Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming.
Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal.
Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal.
PPT Lecture 20 Linear Programming PowerPoint Presentation, free
Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of.
V412. Linear Programming. The Complementary Slackness Theorem. part 2
Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Learn.
complementary slackness theorem with examples YouTube
Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear.
V411. Linear Programming. The Complementary Slackness Theorem. YouTube
Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for.
(PDF) The strict complementary slackness condition in linear fractional
Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear.
1 Complementary Slackness YouTube
Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for.
PPT Duality for linear programming PowerPoint Presentation, free
Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear.
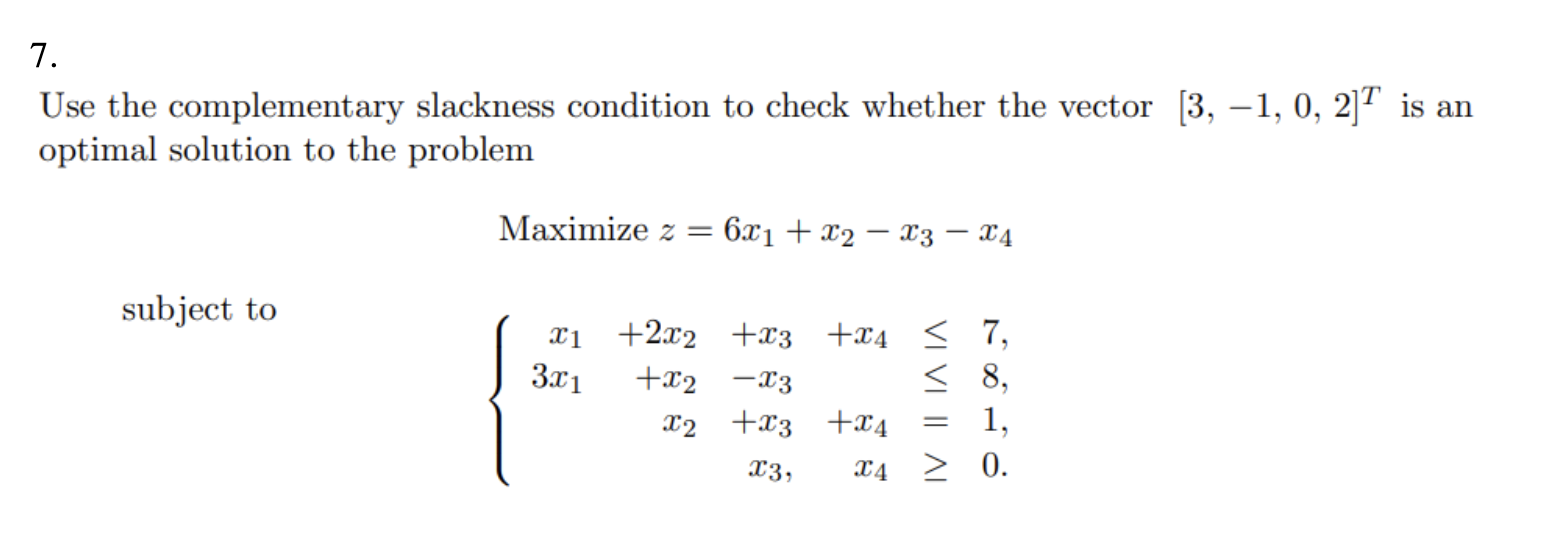
Solved Use the complementary slackness condition to check
Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn.
The Complementary Slackness Theorem (explained with an example dual LP
Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs. Learn.
Complementary Slackness in LP YouTube
Learn how to use complementary slackness conditions to check the optimality of primal and dual solutions in linear programming. Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the.
Learn How To Use Complementary Slackness Conditions To Check The Optimality Of Primal And Dual Solutions In Linear Programming.
Learn how to use normal cones and separation to characterize the normal cone of linear constraints and the kkt conditions for nonlinear. Complementarity slackness can be thought of as a combinatorial optimality condition, where a zero duality gap (equality of the primal. Learn the statement and proof of complementary slackness, a condition that relates primal and dual optimal solutions of linear programs.